how to find the area of a semicircle

Area and Perimeter of a Semicircle

Here we will discuss about the area and perimeter of a semicircle with some example problems.

$Area and Perimeter of a Semicircle$

Area of a semicircle = $\frac{1}{2}$ πr$^{2}$

Perimeter of a semicircle = (π + 2)r

Solved example problems on finding the area and perimeter of a semicircle:

1. Find the area and perimeter of a semicircle of radius 7 cm. (Use π = $\frac{22}{7}$).

Solution:

Given, radius = r = 7 cm.

$Problems on Area and Perimeter of a Semicircle$

Then, area of semicircle = $\frac{1}{2}$ πr$^{2}$

= $\frac{1}{2}$ × $\frac{22}{7}$ × 7$^{2}$ cm$^{2}$

= 11 × 7 cm$^{2}$

= 77 cm$^{2}$

Perimeter of a semicircle = (π + 2)r

= ($\frac{22}{7}$ + 2) × 7 cm

= $\frac{36}{7}$ × 7 cm

= 36 cm

2. Find the area and perimeter of the figure in which PQRS is a square of side 28 cm and STR is a semicircle. (Use π = $\frac{22}{7}$).

$Area and Perimeter of Semicircular Figure$

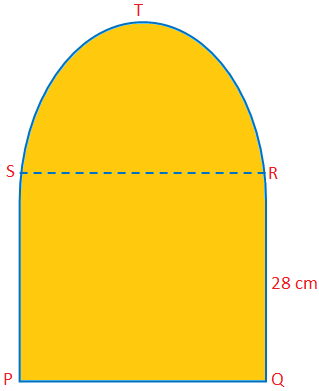
Solution:

The required area = Area of the square PQRS + Area of the semicircle STR

= a$^{2}$ + $\frac{1}{2}$ πr$^{2}$

= 28$^{2}$ cm$^{2}$ + $\frac{1}{2}$ × π × ($\frac{1}{2}$ × 28)$^{2}$ cm$^{2}$

= (28$^{2}$ + $\frac{1}{2}$ × $\frac{22}{7}$ × 14$^{2}$) cm$^{2}$

= (28$^{2}$ + $\frac{1}{2}$ × $\frac{22}{7}$ × 14 × 14) cm$^{2}$

= (28$^{2}$ + 11 × 2 × 14) cm$^{2}$

= (28$^{2}$ + 11 × 28) cm$^{2}$

= 28(28 + 11) cm$^{2}$

= 28 × 39 cm$^{2}$

= 1092 cm$^{2}$

The required perimeter = PQ + PS + QR + semicircular arc STR

= 28 cm + 28 cm + 28 cm + π × ($\frac{1}{2}$ SR)

= 84 cm + $\frac{22}{7}$ × $\frac{1}{2}$ × 28 cm

= 84 cm + 11 × 4 cm

= 84 cm + 44 cm

= 128 cm

9th Grade Math

From Area and Perimeter of a Semicircle to HOME PAGE

Didn't find what you were looking for? Or want to know more information about Math Only Math. Use this Google Search to find what you need.